Decision Support Methods for Tactical Pavement Investment Planning
Chief Technology Officer, Decision Optimization Technology- United States (DOT-US) L.P.
Municipal roads and highway systems are fundamental infrastructure assets. They provide a foundation to the performance of all national economies by sustaining economic development and facilitating social interaction. Preserving and maintaining pavement assets has, therefore, been an important yet challenging task for governments under restricted funding programs. With road assets continually passed down to lower-tier municipalities, the increasing burden of operation and maintenance programs falls to city and county taxpayers. The United States faces a huge infrastructure deficit that will be partially addressed over the next five years with the new $1.2 trillion Infrastructure Investment and Jobs Act — $110 million of which is planned to be allocated to roads, bridges and major transportation projects.
This article discusses some of the more common methods of fund allocation: priority ranking, multicriteria analysis, cost-benefit analysis and true optimization. Each method is compared with others to identify the advantages and disadvantages.
CONDITION-BASED PRIORITY RANKING
 Priority ranking has been suggested and used in many pavement management applications [1]. Using ranking, projects are typically selected in order based on a calculated priority index (PI). Prioritization is generally performed based on agency policies and can range from the subjective opinions of road managers to age- or condition-based ranking methods. Indicators such as pavement condition index (PCI) can be used to prioritize road segments. Other attributes, such as functional class, traffic or minimum service standards, can also be used to determine a PI. After determining a PCI for each road segment, the network is sorted from the highest-priority to the lowest-priority segments. Next, the highest-priority segment is selected, and the required treatment type and its associated cost are determined. If the available budget covers the cost, the segment and its associated treatment are selected.
One of the main problems with using condition-based priority ranking for fund allocation is the resulting “worst roads first” approach. Under this strategy, the most deteriorated roads, which require major rehabilitation treatments, are a huge sink into which the largest portion of municipal road budgets is poured. Unfortunately, this approach makes it impossible to catch up, because while the worst roads are reconstructed at a huge expense, the good roads rapidly deteriorate because of the lack of maintenance and will become the worst roads in a few years. Another problem with priority ranking is the fact that it is performed yearly; therefore, it omits the time dimension of the analysis and cannot analyze the impact of delays on the overall allocation of the budget and network performance.
MULTICRITERIA ANALYSISÂ
Multicriteria analysis (MCA) for decision-making is useful, particularly when dealing with decision-making problems that involve multiple objectives and constraints (criteria). A wide range of MCA methods were developed in the ’80s and ’90s and have become more widely considered in various domains since 2000. MCA methods are diverse in the kinds of problems they address and the techniques they employ. Some examples of the more widely used MCA methods include analytical hierarchy process (AHP) [2, 3], fuzzy AHP [4], multi-attribute utility theory [5] and the Technique for Order of Preference by Similarity to Ideal Solution [6]. In general, MCA methods complement monetary evaluation methods, such as cost-benefit analysis. MCA techniques can help overcome the limitations of human judgment by imposing a systematic and structured approach to evaluate criteria and their relative importance.
Comparisons with condition-based priority ranking suggest that MCA can help to better capture the variations in priority programing and results in more applications of cost-effective, preventive maintenance strategies. MCA is, however, not free of limitations, and care must be taken when employing MCA methods. Although MCA is a better approach compared to priority ranking and a beneficial method in terms of focusing decision-maker attention on developing a formal structure to the decision-making problem, it cannot guarantee that the best possible solutions are achieved.
COST-BENEFIT ANALYSIS
Cost-benefit analysis (CBA) originated from the work of two French engineers, Auguste Cournot and Jules Dupuit in the mid-19th century, who were known as the founding fathers of microeconomics [7]. CBA explicitly determines benefits and costs associated with a project in monetary terms [8, 9]. CBA can be combined with MCA for a more comprehensive analysis, in which CBA assesses the financial aspects of the problem and MCA is used for criteria that cannot be evaluated in monetary terms. Using CBA, an agency can prioritize projects based on the cost-effectiveness or the benefit-to-cost ratio. This is useful for the project-level analyses when a limited number of projects are compared for the upcoming construction season. CBA, however, has several limitations when it comes to effective network-level preservation programing. This is useful at the project-level analyses when a limited number of projects are compared for the upcoming construction season. CBA, however, has several limitations when it comes to effective network-level preservation programing.
Although CBA can get the decision-makers closer to a better solution, it cannot guarantee that the ideal or best solution has been achieved. Considering the monetary value of major infrastructure projects and the large number of investments on renewal projects at the local, municipal and federal levels of government, the difference between an ideal (i.e., optimal) and a good solution can be easily translated into millions of dollars in savings.
OPTIMIZATION
Optimization is branch of science in operations research (OR). OR provides a scientific approach to decision-making that seeks to optimize the performance of a system, usually under conditions requiring the allocation of scarce resources. OR originated during World War II, when the British government recruited scientists from different disciplines to solve the operational problems of the war, such as the deployment of radar and the management of convoy, bombing, anti-submarine and mining operations, which coined the term “operations research.” In the context of optimization, a system can be a collection of interdependent entities that work together to accomplish the goal of the system.
In the context of pavement management, or more generally, asset management, the term “optimization” has been used rather loosely for methods such as incremental cost-benefit analysis, MCA or even priority ranking. These methods, however, cannot be categorized as formal mathematical optimization and are far less effective compared to true optimization. Performing a true optimization analysis for the purpose of the allocation of capital funds, however, presents a complex problem [10]. One of the key challenges associated with the optimization modeling of pavement preservation programs is the exponential increase in solution space as the number of road sections and, consequently, decision variables increase [11].
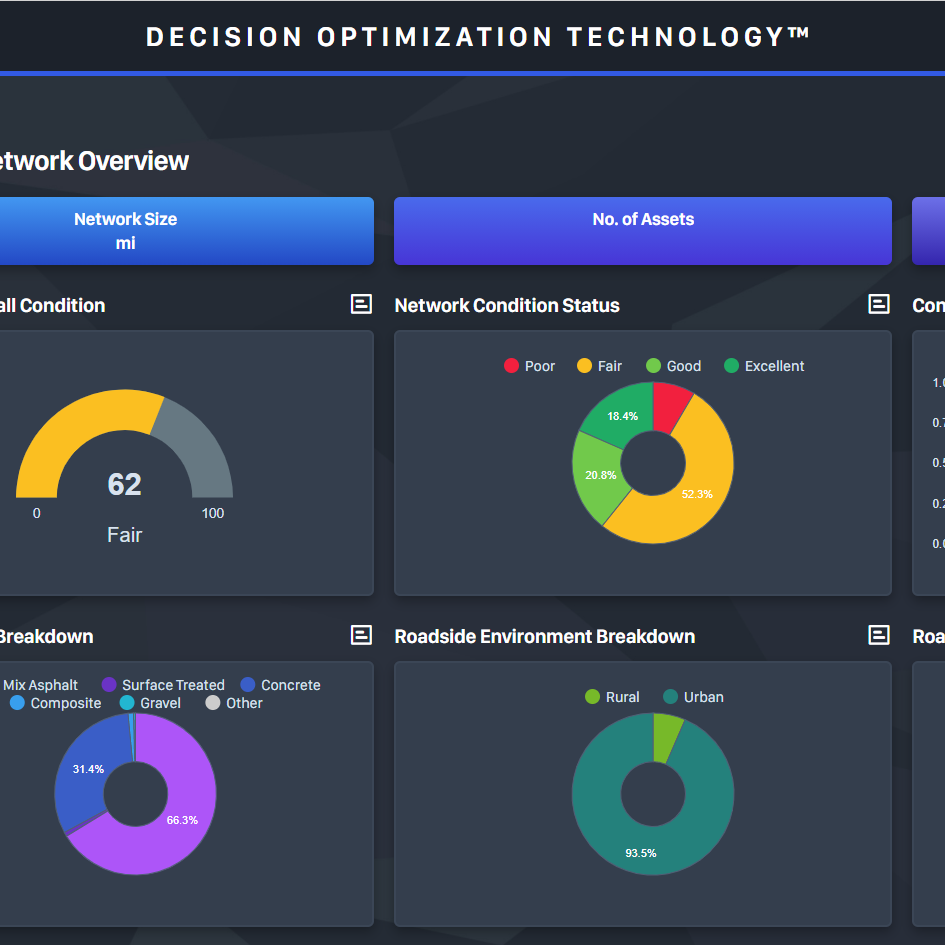
Technologies have emerged that perform true optimization analyses, such as a commercial optimization tool for capital planning called DOT™ (Decision Optimization Technology). DOT™ can optimize large-scale asset management problems to determine the best course of action in terms of timing and selecting an array of preservation treatments that result in the highest investment efficiency while satisfying many constraints regarding serviceability criteria, socio-economic policies, budgetary limits, co-located projects and operational efficiency.
The practical application of optimization in the municipal domain, however, is not free of challenges. Optimizing real-life complex and large-size infrastructure networks is not an easy task. In the context of combinatorial optimization, even a small network with only hundreds of assets is considered a large optimization problem because of the enormous increase in the number of possible combinations and, therefore, solution space. The other challenge associated with the practical application of optimization is the inherent complexity of formulating and developing mathematical optimization models. To overcome these challenges, advanced, powerful optimization technologies are needed that can effectively handle large, complex problems in the municipal domain, while providing a user-friendly interface and an easy-to-understand application framework.
Optimization can achieve higher performance gains over an entire plan when compared with MCA, condition-based priority ranking and CBA. In real-life cases, the expected improvements and the significance of optimization improvements can be more dramatic. New technologies, such as DOT™ software, are becoming available to provide powerful capabilities that can be effectively applied in real-life settings by municipalities, big and small, across North America.
REFERENCES
 [1] Wolters A., Zimmerman K., Schattler, K., & Rietgraf A. (2011). (rep.) Implementing pavement management systems for local agencies — state-of-the-art/state-of-the-practice synthesis (Research Report ICT-11-094-1). Rantoul, IL: Illinois Center for Transportation.
[2] Saaty, T. L. (1990). How to make a decision: The Analytic Hierarchy Process. European Journal of Operational Research, 48(1), 9–26.
[3] Saaty, T. L. (2008), Decision making with the analytic hierarchy process, International Journal of Services Sciences, 1(1), 83–98.
[4] van Laarhoven, P.J.M., & Pedrycz, W. (1983). A fuzzy extension of Saaty’s priority theory. Fuzzy Sets and Systems, 11(1-3), 229–241.
[5] Keeney, R.L., & Raiffa, H. (1993). Decisions with multiple objectives: Preferences and Value Tradeoffs. Cambridge Univ. Press.
[6] Lai, Y., Liu, T., & Hwang, C. (1994). TOPSIS for multi objective decision making. European Journal of Operational Research, 76(3), 486–500.
[7] Arler, F. (2006). (rep.) Ethics and cost-benefit analysis (Research Report 4). Aalborg, Denmark: Aalborg University Department of Development and Planning.
[8] Thoft-Christensen, P. (2011). Infrastructures and life-cycle cost-benefit analysis. Structure and Infrastructure Engineering, 8(5), 507–516.
[9] Fraser, N.M., & Jewkes, E.M. (2013). Engineering economics: Financial decision making for engineers (Fifth ed.). Pearson Canada Inc.
[10] Abaza, K.A. (2007). Expected performance of pavement repair works in a global network optimization model. Journal of Infrastructure Systems, 13(2), 124–134.
[11] Al-Bazi, A., & Dawood, N. (2010). Developing crew allocation system for precast industry using genetic algorithms. Computer-Aided Civil and Infrastructure Engineering, 25(8), 581–595.
Dr. Rashedi serves as the Chief Technology Officer for DOT-US and has been directing the development of asset management software solutions for six years. He has over ten years of experience in optimization modeling and decision support system design in various civil infrastructure domains such as water and wastewater, transportation, structures, bridges, and facilities. He has worked with numerous agencies in North America to assist them with implementation of successful and truly optimized asset management solutions. He has published numerous technical articles in infrastructure asset management and is an Adjunct Professor of Civil Engineering at Ryerson University.
